
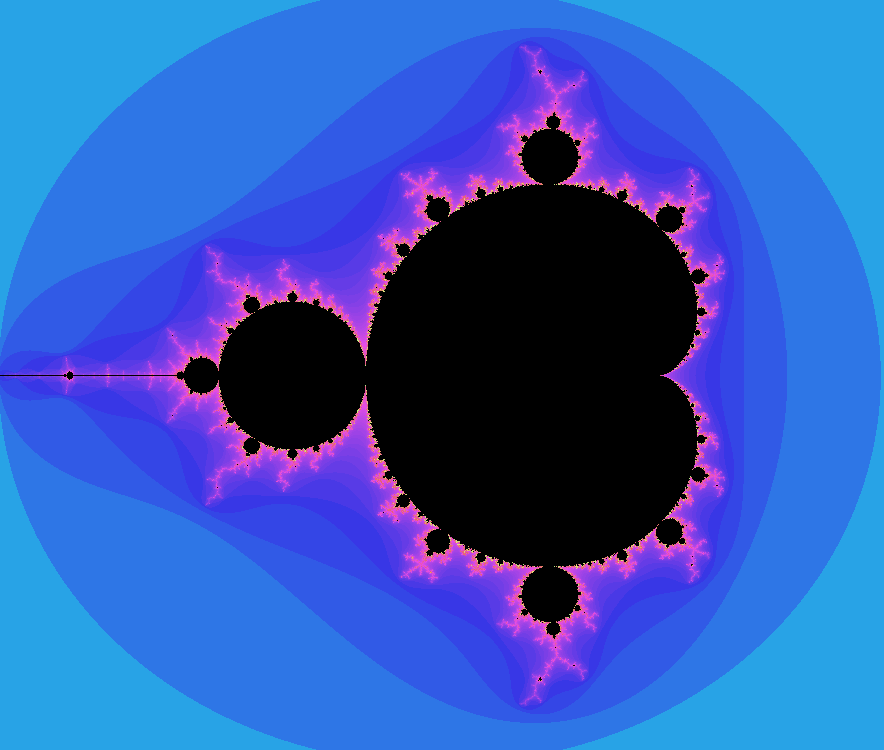

The Mandelbrot set (/ˈmændəlbroʊt, -brɒt/)[1][2] is the set of complex numbers for which the function does not diverge to infinity when iterated from , i.e., for which the sequence , , etc., remains bounded in absolute value.
This set was first defined and drawn by Robert W. Brooks and Peter Matelski in 1978, as part of a study of Kleinian groups.[3] Afterwards, in 1980, Benoit Mandelbrot obtained high quality visualizations of the set while working at IBM's Thomas J. Watson Research Center in Yorktown Heights, New York.
Images of the Mandelbrot set exhibit an elaborate and infinitely complicated boundary that reveals progressively ever-finer recursive detail at increasing magnifications; mathematically, one would say that the boundary of the Mandelbrot set is a fractal curve. The "style" of this recursive detail depends on the region of the set boundary being examined. Mandelbrot set images may be created by sampling the complex numbers and testing, for each sample point whether the sequence goes to infinity. Treating the real and imaginary parts of as image coordinates on the complex plane, pixels may then be coloured according to how soon the sequence crosses an arbitrarily chosen threshold (the threshold has to be at least 2, as -2 is the complex number with the largest magnitude within the set, but otherwise the threshold is arbitrary). If { is held constant and the initial value of is varied instead, one obtains the corresponding Julia set for the point .
The Mandelbrot set has become popular outside mathematics both for its aesthetic appeal and as an example of a complex structure arising from the application of simple rules. It is one of the best-known examples of mathematical visualization, mathematical beauty, and motif.

